M-11 Young's Modulus of Elasticity and Hooke's Law

OBJECTIVE:


 To study the elastic properties of piano wire under tension (Hooke's law and Young's modulus).

APPARATUS:


 Frame for holding the steel wire, optical lever, telescope & support stand for measuring elongation (alternatively, dial gauges), 1 kg slotted masses, micrometer, tape measure.

INTRODUCTION:


 Young's modulus M_Y is the ratio of longitudinal stress to the resultant longitudinal strain:

$$M_Y = \frac{\mbox{stress}}{\mbox{strain}} = \frac{\Delta F/A}{\Delta L/L}$$

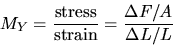
where

Note that

$$\Delta F = \left\{ \frac{M_YA}{L} \right\} \Delta L = k(\Delta L)$$

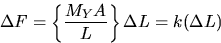
is Hooke's law where k is a constant if the elastic limit is not exceeded.

SUGGESTIONS:

 

1.

First read about the optical lever (appendix 3) and about parallax and focusing a telescope (appendix 4). Also be sure that the frame holding the wire, and the stand holding the telescope are on solid bases. If you use a table, avoid leaning on it. With the telescope check the table sag resulting from leaning on it (least sag when units are near the table legs).

2.

Adjust the height of the platform holding the optical lever so that the long arm of the lever is approximately horizontal.

PROCEDURE:

 

1.

Put a load of three kilograms on the wire to straighten it.

2.

Measure the successive deflections as you increase the load one kilogram at a time up to a total of 10 kg (i.e., 7 kg additional).

3.

Repeat (2) but reducing the load 1 kg at a time.

4.

Convert differences in scale readings to elongations (see appendix 3).

5.

Plot total elongation as abscissa against load in newtons as ordinate. Find the average slope (i.e. $\Delta F/\Delta L = k)$.

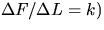6.

Measure A and L; compute Young's modulus. The value for piano wire is $\simeq~20~\times~10^{10}~N/m^2$.

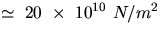QUESTIONS:

 

1.

Piano wire has a tensile strength (breaking stress) of 19 to 23 $\times$ 10⁸ N/m². (The elastic limit may be about 0.7 the breaking stress). Calculate the maximum load your wire could stand. At what load would you pass the elastic limit? Note that the wire fails at a stress which is  100 x less than Young's modulus. Can you understand why the two values are not inconsistent? (Hint: If the stress equaled M_Y, what would be the strain?).

2.

Discuss the sources of error in this experiment and estimate the reliability of your result. Is the accepted value for piano wire steel within the limits you have estimated?

3.

How could you detect slipping of the wire in the chuck during the experiment?

4.

How could you modify the experiment so as to detect and correct for any sagging of the support frame?

5.

Poisson's ratio, 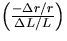$\left(\frac{-\Delta r/r}{\Delta L/L}\right)$, for steel is about 0.3.

Could you notice the decrease in diameter of the wire in this experiment by use of a micrometer caliper? If so, try it.

  Suggested additional experiment: Determine the dependence of elongation on stretching force for a rubber band over a wide range of elongations (up to 3 or more times the unstretched length). Does it obey Hooke's law? Can you suggest reasons for its behavior?